Linux 内核学习笔记系列,GCC 扩展语法和内核数据结构部分,简单介绍 Linux 内核基数树。
基数树简介#
前缀树(字典树)#
该部分内容来源于维基百科。
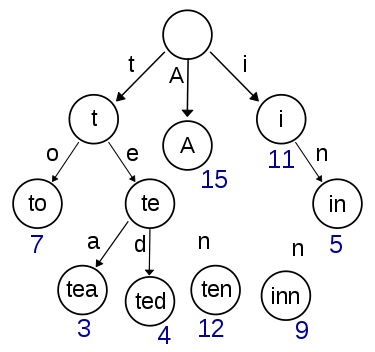
在计算机科学中,trie,又称前缀树或字典树,是一种有序树,用于保存关联数组,其中的键通常是字符串。与二叉查找树不同,键不是直接保存在节点中,而是由节点在树中的位置决定。一个节点的所有子孙都有相同的前缀,也就是这个节点对应的字符串,而根节点对应空字符串。一般情况下,不是所有的节点都有对应的值,只有叶子节点和部分内部节点所对应的键才有相关的值。
在图示中,键标注在节点中,值标注在节点之下。每一个完整的英文单词对应一个特定的整数。Trie可以看作是一个确定有限状态自动机,尽管边上的符号一般是隐含在分支的顺序中的。
键不需要被显式地保存在节点中。图示中标注出完整的单词,只是为了演示trie的原理。
trie中的键通常是字符串,但也可以是其它的结构。trie的算法可以很容易地修改为处理其它结构的有序序列,比如一串数字或者形状的排列。比如,bitwise trie中的键是一串比特,可以用于表示整数或者内存地址。
基数树#
该部分内容来源于维基百科。
在计算机科学中,基数树(也叫基数特里树或压缩前缀树)是一种数据结构,是一种更节省空间的Trie(前缀树),其中作为唯一子节点的每个节点都与其父节点合并,边既可以表示为元素序列又可以表示为单个元素。因此每个内部节点的子节点数最多为基数树的基数r ,其中r为正整数,x为2的幂,x≥1,这使得基数树更适用于对于较小的集合(尤其是字符串很长的情况下)和有很长相同前缀的字符串集合。
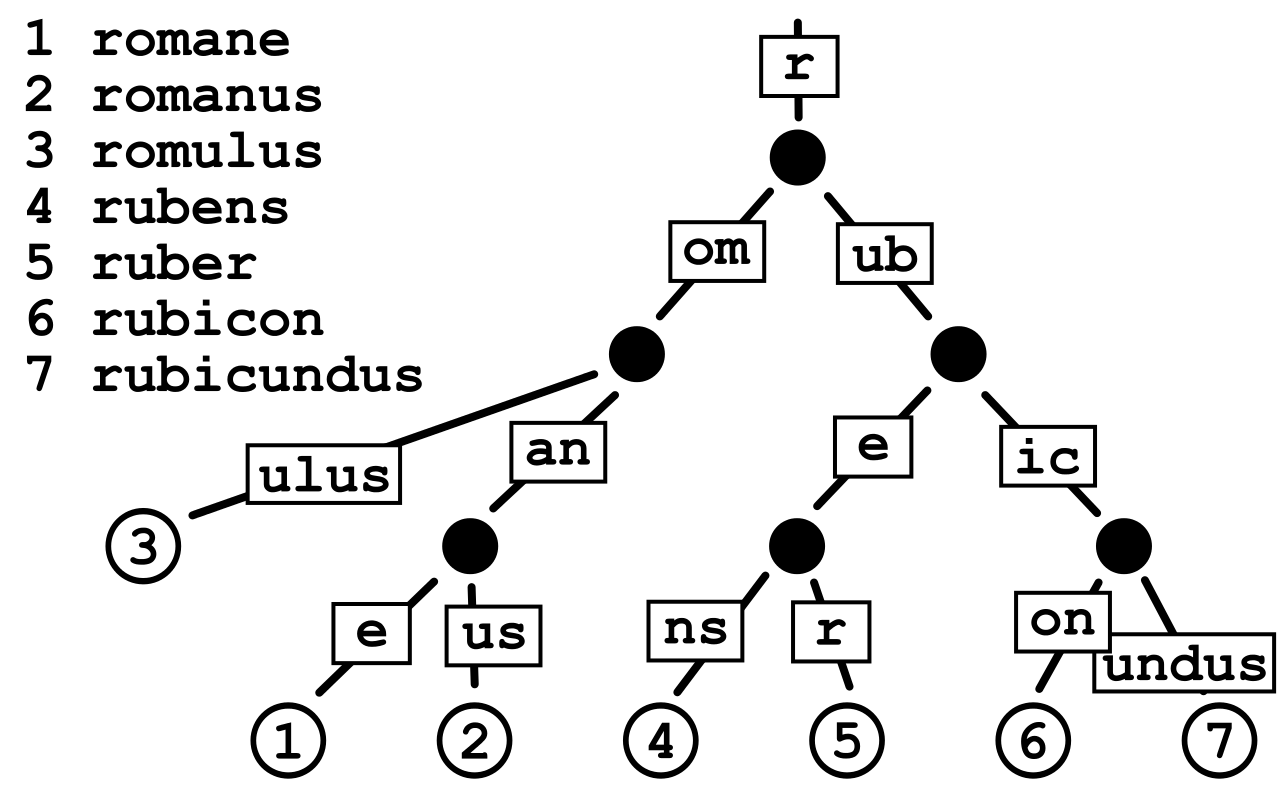
基数树支持插入、删除、查找操作。查找包括完全匹配、前缀匹配、前驱查找、后继查找。所有这些操作都是O(k)复杂度,其中k是所有字符串中最大的长度。
内核基数树的使用#
内核红黑树的实现称为 radix_tree,头文件为 include/linux/radix-tree.h。下面只介绍几个基本的 API 和相关实现。
基数树的创建#
最简单的方式,直接使用宏创建:
1
| RADIX_TREE(name, gfp_mask);
|
或者,也可以手工创建:
1
2
| struct radix_tree_root tree;
INIT_RADIX_TREE(&tree, gfp_mask);
|
基数树的查找#
1
| void *radix_tree_lookup(struct radix_tree_root *root, unsigned long index);
|
该函数在以 root 为根的基数树中查找索引为 index 的内容,返回查找的内容的地址(没找到要查找的内容则返回 NULL)。
1
| unsigned int radix_tree_gang_lookup(struct radix_tree_root *root, void **results, unsigned long first_index, unsigned int max_items);
|
该函数在以 root 为根的基数树中查找非空内容,从索引为 first_index 的结点开始,最多查找 max_items 个非空内容,查找结果放 results 中,返回找到的个数。
基数树的插入#
1
| int radix_tree_insert(struct radix_tree_root *root, unsigned long index, void *item);
|
该函数将内容 item 插入到以 root 为根的基数树中索引为 index 的地方,插入成功返回 0,失败返回错误值。
基数树的删除#
1
| void *radix_tree_delete(struct radix_tree_root *root, unsigned long index);
|
该函数将索引为 index 的内容从以 root 为根的基数树中删除,返回删除的内容的地址(要删除的内容不存在则返回 NULL)。
内核基数树的的实现#
内核基数树的实现均位于 lib/radix-tree.c 和 include/linux/radix-tree.h。我只求会用 API,具体实现的原理要参考数据结构书籍,下面也只贴出部分核心代码。
基数树的定义#
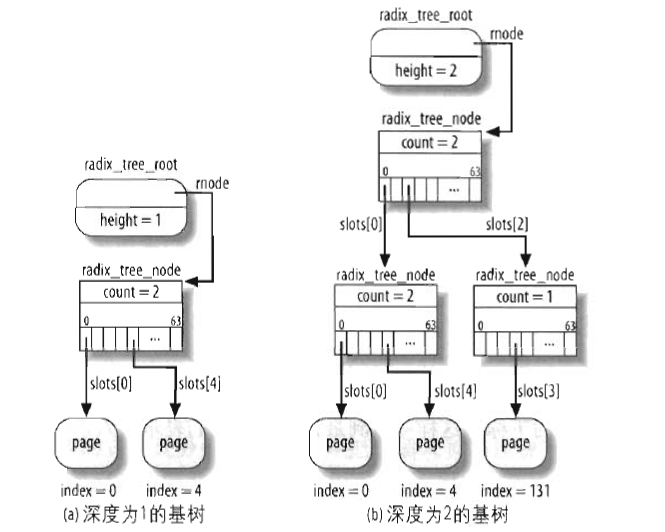
根的定义如下:
1
2
3
4
5
6
| /* root tags are stored in gfp_mask, shifted by __GFP_BITS_SHIFT */
struct radix_tree_root {
unsigned int height;
gfp_t gfp_mask;
struct radix_tree_node *rnode;
};
|
结点的定义如下:
1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
| #define RADIX_TREE_MAX_TAGS 2
#ifdef __KERNEL__
#define RADIX_TREE_MAP_SHIFT (CONFIG_BASE_SMALL ? 4 : 6)
#else
#define RADIX_TREE_MAP_SHIFT 3 /* For more stressful testing */
#endif
#define RADIX_TREE_MAP_SIZE (1UL << RADIX_TREE_MAP_SHIFT)
#define RADIX_TREE_TAG_LONGS \
((RADIX_TREE_MAP_SIZE + BITS_PER_LONG - 1) / BITS_PER_LONG)
struct radix_tree_node {
unsigned int height; /* Height from the bottom */
unsigned int count;
struct rcu_head rcu_head;
void *slots[RADIX_TREE_MAP_SIZE];
unsigned long tags[RADIX_TREE_MAX_TAGS][RADIX_TREE_TAG_LONGS];
};
|
其中,BITS_PER_LONG 是体系结构相关的,指该体系结构下 long 占多少位,该宏在 32 位系统下通常是 32,64 位系统下通常是 64。
基数树创建的实现#
1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
| #define RADIX_TREE_INIT(mask) { \
.height = 0, \
.gfp_mask = (mask), \
.rnode = NULL, \
}
#define RADIX_TREE(name, mask) \
struct radix_tree_root name = RADIX_TREE_INIT(mask)
#define INIT_RADIX_TREE(root, mask) \
do { \
(root)->height = 0; \
(root)->gfp_mask = (mask); \
(root)->rnode = NULL; \
} while (0)
|
辅助函数/宏的实现#
1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
| #define RADIX_TREE_INDIRECT_PTR 1
static inline void *radix_tree_indirect_to_ptr(void *ptr)
{
return (void *)((unsigned long)ptr & ~RADIX_TREE_INDIRECT_PTR);
}
static inline int radix_tree_is_indirect_ptr(void *ptr)
{
return (int)((unsigned long)ptr & RADIX_TREE_INDIRECT_PTR);
}
/*
* Return the maximum key which can be store into a
* radix tree with height HEIGHT.
*/
static inline unsigned long radix_tree_maxindex(unsigned int height)
{
return height_to_maxindex[height];
}
|
对于前两个函数,由于地址总是对齐的,不管哪种体系结构,地址的最后一位总是 0。所以可以利用这一位,存储该结点是直接结点(指针直接指向内容)还是间接结点(指针指向其他树的结点)。
基数树的查找的实现#
1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32
33
34
35
36
37
38
39
40
41
42
43
44
45
46
47
48
49
50
51
52
53
54
55
56
57
58
59
60
| #define RADIX_TREE_MAP_MASK (RADIX_TREE_MAP_SIZE-1)
/*
* is_slot == 1 : search for the slot.
* is_slot == 0 : search for the node.
*/
static void *radix_tree_lookup_element(struct radix_tree_root *root,
unsigned long index, int is_slot)
{
unsigned int height, shift;
struct radix_tree_node *node, **slot;
node = rcu_dereference_raw(root->rnode);
if (node == NULL)
return NULL;
if (!radix_tree_is_indirect_ptr(node)) {
if (index > 0)
return NULL;
return is_slot ? (void *)&root->rnode : node;
}
node = radix_tree_indirect_to_ptr(node);
height = node->height;
if (index > radix_tree_maxindex(height))
return NULL;
shift = (height-1) * RADIX_TREE_MAP_SHIFT;
do {
slot = (struct radix_tree_node **)
(node->slots + ((index>>shift) & RADIX_TREE_MAP_MASK));
node = rcu_dereference_raw(*slot);
if (node == NULL)
return NULL;
shift -= RADIX_TREE_MAP_SHIFT;
height--;
} while (height > 0);
return is_slot ? (void *)slot:node;
}
/**
* radix_tree_lookup - perform lookup operation on a radix tree
* @root: radix tree root
* @index: index key
*
* Lookup the item at the position @index in the radix tree @root.
*
* This function can be called under rcu_read_lock, however the caller
* must manage lifetimes of leaf nodes (eg. RCU may also be used to free
* them safely). No RCU barriers are required to access or modify the
* returned item, however.
*/
void *radix_tree_lookup(struct radix_tree_root *root, unsigned long index)
{
return radix_tree_lookup_element(root, index, 0);
}
EXPORT_SYMBOL(radix_tree_lookup);
|
radix_tree_lookup() 用于查找单个内容,RCU 相关的内容还没看,这里先无视(见 TODO 列表)。
1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32
33
34
35
36
37
38
39
40
41
42
43
44
45
46
47
48
49
50
51
52
53
54
55
56
57
58
59
60
61
62
63
64
65
66
67
68
69
70
71
72
73
74
75
76
77
78
79
80
81
82
83
84
85
86
87
88
89
90
91
92
93
94
95
96
97
98
99
100
101
102
103
104
105
106
107
108
109
110
111
112
113
114
115
116
| static unsigned int
__lookup(struct radix_tree_node *slot, void ***results, unsigned long index,
unsigned int max_items, unsigned long *next_index)
{
unsigned int nr_found = 0;
unsigned int shift, height;
unsigned long i;
height = slot->height;
if (height == 0)
goto out;
shift = (height-1) * RADIX_TREE_MAP_SHIFT;
for ( ; height > 1; height--) {
i = (index >> shift) & RADIX_TREE_MAP_MASK;
for (;;) {
if (slot->slots[i] != NULL)
break;
index &= ~((1UL << shift) - 1);
index += 1UL << shift;
if (index == 0)
goto out; /* 32-bit wraparound */
i++;
if (i == RADIX_TREE_MAP_SIZE)
goto out;
}
shift -= RADIX_TREE_MAP_SHIFT;
slot = rcu_dereference_raw(slot->slots[i]);
if (slot == NULL)
goto out;
}
/* Bottom level: grab some items */
for (i = index & RADIX_TREE_MAP_MASK; i < RADIX_TREE_MAP_SIZE; i++) {
index++;
if (slot->slots[i]) {
results[nr_found++] = &(slot->slots[i]);
if (nr_found == max_items)
goto out;
}
}
out:
*next_index = index;
return nr_found;
}
/**
* radix_tree_gang_lookup - perform multiple lookup on a radix tree
* @root: radix tree root
* @results: where the results of the lookup are placed
* @first_index: start the lookup from this key
* @max_items: place up to this many items at *results
*
* Performs an index-ascending scan of the tree for present items. Places
* them at *@results and returns the number of items which were placed at
* *@results.
*
* The implementation is naive.
*
* Like radix_tree_lookup, radix_tree_gang_lookup may be called under
* rcu_read_lock. In this case, rather than the returned results being
* an atomic snapshot of the tree at a single point in time, the semantics
* of an RCU protected gang lookup are as though multiple radix_tree_lookups
* have been issued in individual locks, and results stored in 'results'.
*/
unsigned int
radix_tree_gang_lookup(struct radix_tree_root *root, void **results,
unsigned long first_index, unsigned int max_items)
{
unsigned long max_index;
struct radix_tree_node *node;
unsigned long cur_index = first_index;
unsigned int ret;
node = rcu_dereference_raw(root->rnode);
if (!node)
return 0;
if (!radix_tree_is_indirect_ptr(node)) {
if (first_index > 0)
return 0;
results[0] = node;
return 1;
}
node = radix_tree_indirect_to_ptr(node);
max_index = radix_tree_maxindex(node->height);
ret = 0;
while (ret < max_items) {
unsigned int nr_found, slots_found, i;
unsigned long next_index; /* Index of next search */
if (cur_index > max_index)
break;
slots_found = __lookup(node, (void ***)results + ret, cur_index,
max_items - ret, &next_index);
nr_found = 0;
for (i = 0; i < slots_found; i++) {
struct radix_tree_node *slot;
slot = *(((void ***)results)[ret + i]);
if (!slot)
continue;
results[ret + nr_found] = rcu_dereference_raw(slot);
nr_found++;
}
ret += nr_found;
if (next_index == 0)
break;
cur_index = next_index;
}
return ret;
}
EXPORT_SYMBOL(radix_tree_gang_lookup);
|
radix_tree_gang_lookup() 用于查找多个内容。
其他查找函数都是同理,只是查找的东西,比如查找 slot 和 tag 等。
基数树的插入的实现#
1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32
33
34
35
36
37
38
39
40
41
42
43
44
45
46
47
48
49
50
51
52
53
54
55
56
57
58
59
60
61
62
63
64
65
66
67
68
69
70
71
72
73
74
75
76
77
78
79
80
81
82
83
84
85
86
87
88
89
90
91
92
93
94
95
96
97
98
99
100
101
102
103
104
105
106
107
108
109
110
111
112
113
114
115
116
117
118
119
120
121
122
123
124
125
126
127
128
129
130
131
132
133
134
135
136
137
138
139
140
141
142
143
144
145
146
147
148
149
150
151
| static inline gfp_t root_gfp_mask(struct radix_tree_root *root)
{
return root->gfp_mask & __GFP_BITS_MASK;
}
/*
* This assumes that the caller has performed appropriate preallocation, and
* that the caller has pinned this thread of control to the current CPU.
*/
static struct radix_tree_node *
radix_tree_node_alloc(struct radix_tree_root *root)
{
struct radix_tree_node *ret = NULL;
gfp_t gfp_mask = root_gfp_mask(root);
if (!(gfp_mask & __GFP_WAIT)) {
struct radix_tree_preload *rtp;
/*
* Provided the caller has preloaded here, we will always
* succeed in getting a node here (and never reach
* kmem_cache_alloc)
*/
rtp = &__get_cpu_var(radix_tree_preloads);
if (rtp->nr) {
ret = rtp->nodes[rtp->nr - 1];
rtp->nodes[rtp->nr - 1] = NULL;
rtp->nr--;
}
}
if (ret == NULL)
ret = kmem_cache_alloc(radix_tree_node_cachep, gfp_mask);
BUG_ON(radix_tree_is_indirect_ptr(ret));
return ret;
}
/*
* Extend a radix tree so it can store key @index.
*/
static int radix_tree_extend(struct radix_tree_root *root, unsigned long index)
{
struct radix_tree_node *node;
unsigned int height;
int tag;
/* Figure out what the height should be. */
height = root->height + 1;
while (index > radix_tree_maxindex(height))
height++;
if (root->rnode == NULL) {
root->height = height;
goto out;
}
do {
unsigned int newheight;
if (!(node = radix_tree_node_alloc(root)))
return -ENOMEM;
/* Increase the height. */
node->slots[0] = radix_tree_indirect_to_ptr(root->rnode);
/* Propagate the aggregated tag info into the new root */
for (tag = 0; tag < RADIX_TREE_MAX_TAGS; tag++) {
if (root_tag_get(root, tag))
tag_set(node, tag, 0);
}
newheight = root->height+1;
node->height = newheight;
node->count = 1;
node = radix_tree_ptr_to_indirect(node);
rcu_assign_pointer(root->rnode, node);
root->height = newheight;
} while (height > root->height);
out:
return 0;
}
/**
* radix_tree_insert - insert into a radix tree
* @root: radix tree root
* @index: index key
* @item: item to insert
*
* Insert an item into the radix tree at position @index.
*/
int radix_tree_insert(struct radix_tree_root *root,
unsigned long index, void *item)
{
struct radix_tree_node *node = NULL, *slot;
unsigned int height, shift;
int offset;
int error;
BUG_ON(radix_tree_is_indirect_ptr(item));
/* Make sure the tree is high enough. */
if (index > radix_tree_maxindex(root->height)) {
error = radix_tree_extend(root, index);
if (error)
return error;
}
slot = radix_tree_indirect_to_ptr(root->rnode);
height = root->height;
shift = (height-1) * RADIX_TREE_MAP_SHIFT;
offset = 0; /* uninitialised var warning */
while (height > 0) {
if (slot == NULL) {
/* Have to add a child node. */
if (!(slot = radix_tree_node_alloc(root)))
return -ENOMEM;
slot->height = height;
if (node) {
rcu_assign_pointer(node->slots[offset], slot);
node->count++;
} else
rcu_assign_pointer(root->rnode,
radix_tree_ptr_to_indirect(slot));
}
/* Go a level down */
offset = (index >> shift) & RADIX_TREE_MAP_MASK;
node = slot;
slot = node->slots[offset];
shift -= RADIX_TREE_MAP_SHIFT;
height--;
}
if (slot != NULL)
return -EEXIST;
if (node) {
node->count++;
rcu_assign_pointer(node->slots[offset], item);
BUG_ON(tag_get(node, 0, offset));
BUG_ON(tag_get(node, 1, offset));
} else {
rcu_assign_pointer(root->rnode, item);
BUG_ON(root_tag_get(root, 0));
BUG_ON(root_tag_get(root, 1));
}
return 0;
}
EXPORT_SYMBOL(radix_tree_insert);
|
基数树的删除的实现#
1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32
33
34
35
36
37
38
39
40
41
42
43
44
45
46
47
48
49
50
51
52
53
54
55
56
57
58
59
60
61
62
63
64
65
66
67
68
69
70
71
72
73
74
75
76
77
78
79
80
81
82
83
84
85
86
87
88
89
90
91
92
93
94
95
96
97
98
99
100
101
102
103
104
105
106
107
108
109
110
111
112
113
114
115
116
117
118
119
120
121
122
123
124
125
126
127
128
129
130
131
132
133
134
135
136
137
138
139
140
| /**
* radix_tree_shrink - shrink height of a radix tree to minimal
* @root radix tree root
*/
static inline void radix_tree_shrink(struct radix_tree_root *root)
{
/* try to shrink tree height */
while (root->height > 0) {
struct radix_tree_node *to_free = root->rnode;
void *newptr;
BUG_ON(!radix_tree_is_indirect_ptr(to_free));
to_free = radix_tree_indirect_to_ptr(to_free);
/*
* The candidate node has more than one child, or its child
* is not at the leftmost slot, we cannot shrink.
*/
if (to_free->count != 1)
break;
if (!to_free->slots[0])
break;
/*
* We don't need rcu_assign_pointer(), since we are simply
* moving the node from one part of the tree to another. If
* it was safe to dereference the old pointer to it
* (to_free->slots[0]), it will be safe to dereference the new
* one (root->rnode).
*/
newptr = to_free->slots[0];
if (root->height > 1)
newptr = radix_tree_ptr_to_indirect(newptr);
root->rnode = newptr;
root->height--;
radix_tree_node_free(to_free);
}
}
static inline void
radix_tree_node_free(struct radix_tree_node *node)
{
call_rcu(&node->rcu_head, radix_tree_node_rcu_free);
}
/**
* radix_tree_delete - delete an item from a radix tree
* @root: radix tree root
* @index: index key
*
* Remove the item at @index from the radix tree rooted at @root.
*
* Returns the address of the deleted item, or NULL if it was not present.
*/
void *radix_tree_delete(struct radix_tree_root *root, unsigned long index)
{
/*
* The radix tree path needs to be one longer than the maximum path
* since the "list" is null terminated.
*/
struct radix_tree_path path[RADIX_TREE_MAX_PATH + 1], *pathp = path;
struct radix_tree_node *slot = NULL;
struct radix_tree_node *to_free;
unsigned int height, shift;
int tag;
int offset;
height = root->height;
if (index > radix_tree_maxindex(height))
goto out;
slot = root->rnode;
if (height == 0) {
root_tag_clear_all(root);
root->rnode = NULL;
goto out;
}
slot = radix_tree_indirect_to_ptr(slot);
shift = (height - 1) * RADIX_TREE_MAP_SHIFT;
pathp->node = NULL;
do {
if (slot == NULL)
goto out;
pathp++;
offset = (index >> shift) & RADIX_TREE_MAP_MASK;
pathp->offset = offset;
pathp->node = slot;
slot = slot->slots[offset];
shift -= RADIX_TREE_MAP_SHIFT;
height--;
} while (height > 0);
if (slot == NULL)
goto out;
/*
* Clear all tags associated with the just-deleted item
*/
for (tag = 0; tag < RADIX_TREE_MAX_TAGS; tag++) {
if (tag_get(pathp->node, tag, pathp->offset))
radix_tree_tag_clear(root, index, tag);
}
to_free = NULL;
/* Now free the nodes we do not need anymore */
while (pathp->node) {
pathp->node->slots[pathp->offset] = NULL;
pathp->node->count--;
/*
* Queue the node for deferred freeing after the
* last reference to it disappears (set NULL, above).
*/
if (to_free)
radix_tree_node_free(to_free);
if (pathp->node->count) {
if (pathp->node ==
radix_tree_indirect_to_ptr(root->rnode))
radix_tree_shrink(root);
goto out;
}
/* Node with zero slots in use so free it */
to_free = pathp->node;
pathp--;
}
root_tag_clear_all(root);
root->height = 0;
root->rnode = NULL;
if (to_free)
radix_tree_node_free(to_free);
out:
return slot;
}
EXPORT_SYMBOL(radix_tree_delete);
|


